
You may have heard the term “stacking fault.” Perhaps you’ve realized that the FCC and HCP crystal structures have the same atomic density. If you’ve studied further, you might even know that FCC and HCP are both “close-packed” structures–but you may not know why that is. I would guess that you only know these two close-packed crystals; but in principle, there are an infinite number of close-packed crystals.
Close-packed crystal structures are atomic arrangements where a single type of atom is arranged to achieve the highest possible Atomic Packing Factor (APF) of 74%. Close-packed crystals must have close-packed, hexagonal 2D planes; the ways these planes are stacked is called “Stacking Order” and is the distinguishing characteristic between close-packed structures.
Let’s explain stacking order by building some crystals.
Outline
The 2D Close-packed Plane
In two dimensions, there is only one way to arrange spheres to achieve maximum area density. That arrangement is in a hexagonal pattern, like the hexagonal Bravais Lattice.
One crude way to estimate a structure’s stability is to calculate its Atomic Packing Factor (APF). I’ve written a comprehensive article about APF, but it essentially calculates the ratio of space that is filled with atoms compared to empty void.
Generally speaking, the more space that can be filled with atoms, the more stable a structure is.
Because of this, most materials form a hexagonal lattice if they are made in layers 1-atom thick, like graphene.
If you wanted to make a 3D structure that had the highest APF (i.e. a close-packed structure), a good place to start would be by stacking multiple of these 2D close packed planes. This is the origin of “stacking order.”
Stacking Order
Imagine a close-packed hexagonal plane.
Now, stack another of these planes on top of the first one. Of course, you won’t have an atom directly stacked on top of another–that’s hardly more stable than stacking two basketballs on top of each other.
So your next plane of atoms needs to fit in the gaps of your first layer, layer A. As you can see, there are 6 gaps. If you placed another close packed layer on top of layer A, you’d only be able to fill 3 of those gaps–so we can label 3 of them position B, and 3 of them position C.
So let’s assume we stack layer 1 as A and layer 2 as B. How should we stack layer 3? Now, it’s possible to have another layer of A, or a new layer: C.
This is stacking order (a mistake in stacking order is called a stacking fault).
If your stacking order was AB-AB-AB, the result is a Hexagonal Close-Packed (HCP) crystal structure.
If your stacking order was ABC-ABC, the result is a Face-Centered Cubic (FCC) crystal structure, although you need to look at it from a different angle to see.
HCP and FCC are two of the most common crystal structures. However, there are technically an infinite number of close-packed crystal structures. Any crystal that used stacks of close-packed planes would necessarily be close-packed.
That means that you could pick any stacking order, and it would be a valid crystal. The only rule is that you can’t have a letter repeat twice as in ABBA-ABBA.
ABAC-ABAC would be a true close-packed structure, as would ABCB-ABCB, or even ABABABABC-ABABABABC.
In fact, there is another common crystal structure, which I call Rhombohedral Close-Packed (more popular is “Sm-type”) that occurs in several elements including samarium, gadolinium, terbium, and more. This Sm-type structure has stacking order ABABCBCAC-ABABCBCAC.
Yep, that’s 9 layers before it repeats, which is why it only has rhombohedral symmetry.
In principle, it’s possible that you could have infinitely large unit cells by having a stacking order like ABABABABABABABABABABABABC-ABABABABABABABABABABABABC, with a dozen AB pairs and one C in the 25th spot–however, in real life the interatomic forces don’t really extend so far, so you couldn’t reliably count on a C layer in every 25th position. Instead, you’d just call this an HCP with a high probability of stacking faults.
Stacking Faults
A stacking fault is a type of crystallographic defect, where a plane of atoms is in the wrong position. For example, you know that FCC has stacking order ABC-ABC-ABC-ABC. A stacking fault could be an extra plane of atoms, such as ABC-B-ABC-ABC, or a removed plane of atoms, such as ABC-BC-ABC-ABC. (I added dashes and bold text to make this easier to read, but the dashes don’t represent anything within the crystal.)
Stacking faults are one type of 2D defect, which relates closely to close-packed structures. Twin boundaries and antiphase boundaries also occur in close-packed structures.
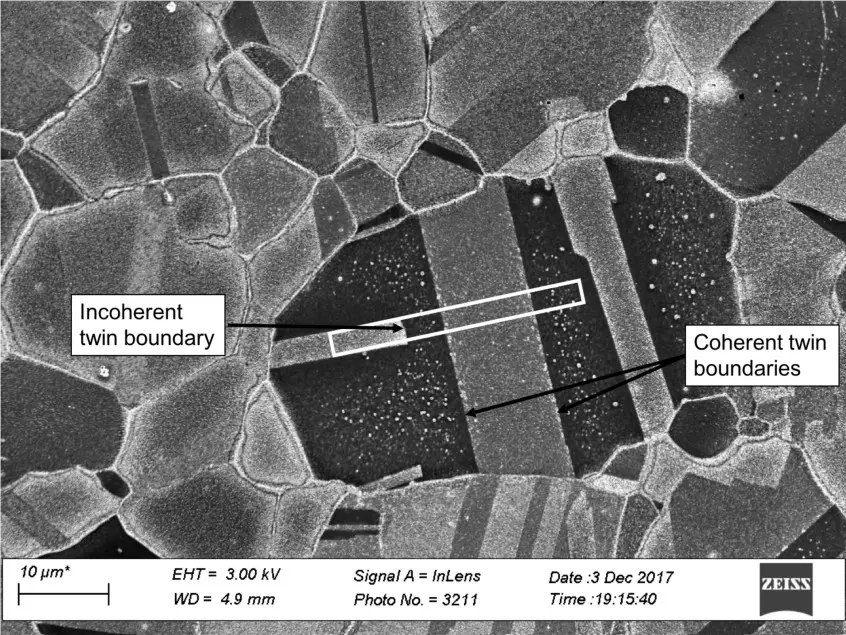
Twin Boundary
A twin boundary is a reversal in the crystal lattice, such as ABC | BA-CBA-CBA. (The | represents the point of the twin boundary, where the stacking order reverses).
Twin boundaries are an important defect in some materials, such as tin and nitinol. Twin boundaries are also quite obvious to see in the microstructure.
Antiphase Boundary
An antiphase boundary is actually not unique to close-packed structures, but you can still use close-packed planes to illustrate them.
In an antiphase region, a portion of material shifts over. For example: ABC-A|ABC-ABC-ABC|C-ABC. The bolded portion has shifted over by one spot, which creates antiphase boundaries so that two of the same layers are stacked on top of each other.
This is energetically unfavorable, like trying to balance two spheres on top of each other. The creation of antiphase boundaries is one reason why superalloys are so strong, and why ceramics are so brittle.
Types of Close-Packed Crystal Structures
There are 4 close-packed crystal structures that occur in pure elements at standard temperature and pressure
| Close-packed Crystal Structure | Type of Packing |
| Face-Centered Cubic (FCC) | 3 layer ABC-ABC packing |
| Hexagonal Close-Packed (HCP) | 2 layer AB-AB packing |
| Double Hexagonal Close-Packed (DHCP) | 4 layer ABAC-ABAC packing |
| Rhombohedral Close Packed (Sm-type) | 9 layer ABABCBCAC-ABABCBCAC packing |
There are also compounds that exhibit close-packed structures, and when I discuss notation you will see more structures that can exist. Remember that there are technically an infinite number of possible structures, but only about 6 make sense when considering short range interatomic forces.
How to Write Notation for Close-Packed Structures
If you’re doing a PhD in crystallography and you need to learn different types of notation, Krishna and Pandey have an excellent article which you can find here.
For those who aren’t doing their PhD in crystallography, you can click to expand a summary of these notation methods.
Notation Comparison Table
To start, here is a table relating the Ramsdell, ABC, Zhadanov, and h-c notation styles.
| ABC sequence | Ramsdell notation | Zhdanov number | h-c notation | Example |
| AB | 2H | (11) | h | HCP |
| ABC | 3C | ∞ | c | FCC |
| ABCB | 4H | (22) | hc | DHCP |
| ABCACB | 6H1 | (33) | hcc | SiC |
| ABCBAB | 6H2 | (2211) | hchchh | — |
| ABACACBCB | 9R | (12) | hhc | Close-packed rhombohedral |
ABC Notation
As you can tell, I prefer the ABC notation. Simply designate your first layer as A, the second as B, and if a third one comes up, that one is C. If using compounds such as CdI2, you can write: AγBCαB, where the greek letters represent secondary atomic layers. In the case of CdI2, γ is a layer of Cd atoms which has the same position as iodine’s C-layer. Similarly α is a layer of Cd with the same position as iodine’s A-layer.
Note that since the crystal structure is fixed, only the information ABCB is needed to describe CdI2, and it falls in the Ramsdell 4H category.
Ramsdell Notation
In scientific papers, I also see the Ramsdell notation. This notation system specifies the number of planes until the structure repeats, and also tells the symmetry relationship. H is hexagonal, C is cubic, and R is rhombohedral.
Zhdanov Number Notation
The Zhdanov number is the most complicated in my opinion, but it is the favorite system of Krishna and Pandey. The idea is that going from atomic layer A–>B implies a rotation in one direction (let’s arbitrarily choose clockwise), and going from atomic layer B–>C or C–>A would imply a rotation in that same clockwise direction. In contrast, going from B–>A is a rotation in the counterclockwise direction.
The Zhadanov number counts the consecutive clockwise and counterclockwise rotations until the original symmetry is reached. For example, AB-AB stacking is 1 clockwise rotation from A–>B, then 1 counterclockwise rotation from B–>C, and you have the original position with Zhadanov number one and one (11). The ABC-ABC stacking involves only clockwise rotations, which repeat infinitely with no counterclockwise rotations. Thus, the Zhadanov number is infinity (∞).
The ABACACBCB-ABACACBCB structure is made of one clockwise rotation, two counterclockwise rotations, and then the relative rotations repeat. Although it takes 9 layers for the absolute atomic positions to repeat, the Zhadanov number clearly shows the short-range order in the (12) structure.
h-c Notation
The h-c notation considers the atoms above and below any particular layer, so I like to think of this as “sandwich notation.” If those layers are the same, then the sandwiched layer is called “h.” If those layers are different, the sandwiched layer is called “c.”
For AB packing, the atomic layers look like ABABABAB….As you can see, every A layer has two B layers sandwiching it, and every B layer has two A layers sandwiching it. Thus, it’s just “h.”
Similarly, for ABC packing the first layer is sandwiched between C and B (which are different), so the first layer is “c.” The second layer is sandwiched between A and C, the third layer is sandwiched between B and A, and you see that ever single layer is sandwiched between two different layers, so the crystal is overall just “c.”
For ABCB packing, the first layer is sandwiched between two B layers, so “h.” The second layer is between A and C, so “c”. The third layer is between two B layers, so “h.” The 4th layer is between C and A, so “c.” The “hc” pattern keeps continuing, so that’s the overall crystal name according to h-c notation.
SiC Prototypes
SiC and ZnS can actually display many different stacking patterns. Here is a chart of these known SiC structures from Krishna and Pandey [2].
| Polytype | Structure (Zhdanov sequence) | Polytype | Structure (Zhdanov sequence) |
| 2H | 11 | 54H | (33)6323334 |
| 3C | ∞ | 57H | (23)93333 |
| 4H | 22 | 57R | (33)234 |
| 6H | 33 | 69R1 | (33)332 |
| 8H | 44 | 69R2 | 33322334 |
| 10H | 3322 | 75R1 | (33)334 |
| 14H | (22)233 | 75R2 | (32)3(23)2 |
| 15R | 23 | 81H | (33)535(33)634 |
| 16H1 | (33)222 | 84R | (33)3(32)2 |
| 16H2 | 332332 | 87R | (33)432 |
| 18H | (22)333 | 90R | (23)43322 |
| 19H | (23)322 | 96R | (33)33434 |
| 20H | (22)344 | 99R | (33)43222 |
| 21H | 333534 | 105R | (33)532 |
| 21R | 34 | 111R | (33)534 |
| 24R | 35 | 120R | (22)523222333 |
| 27H | (33)2(23)3 | 123R | (33)632 |
| 27R | 2223 | 141R | (33)732 |
| 33R | 3332 | 147R | (3332)432 |
| 33H1 | (33)2353334 | 159R | (33)832 |
| 33H2 | (33)3(23)3 | 168R | (23)1033 |
| 36H1 | (33)232(33)234 | 174R | (33)66(33)54 |
| 36H2 | (33)43234 | 189R | (34)843 |
| 39H | (33)232(33)3(32)2 | 222R | (33)634(33)434 |
| 39R | 3334 | 267R | (23)1722 |
| 45R | 232332 | 273R | (23)1733 |
| 51R1 | (33)232 | 303R | (33)1632 |
| 51R2 | (22)323 | 393R | (33)2132 |
Related Questions
Can you have a close-packed structure made of multiple elements?
Yes.
The term “close-packed” assumes that you have an infinite number of perfect spheres, and want a repeating arrangement to maximize their packing density. Since atoms can usually be approximated as spheres, we generally think close-packed structures are made of a single element. However, if you have atoms with similar atomic radii, they can also form close-packed structures.
Examples of close-packed compounds include SiC, ZnS, CdI2, PbI2, AgI and GaSe [2].
Technically, there could be close-packed structures with different atomic radii, as there would be a way to pack those atoms most-densely. However, proving that such a structure is truly close-packed isn’t easy, and these structures would not resemble the “traditional” close-packed structures like FCC or L12.
How close packed is Close Packed?
You’ll never see a pure material with exactly packing. In addition to defects which lower the packing density, atoms are not truly incompressible spheres and they don’t have the exact positions that you calculate in ideal crystal cells.
For example, you can follow the geometric calculations to conclude that HCP crystals should have a c/a ratio of , or 1.633. However, real HCP materials do not have this idea c/a ratio.
| Metal | Lattice constant a (nm) | Lattice constant c (nm) | Atomic radius R (nm) | c/a ratio | % deviation from ideality |
| Cadmium | 0.2973 | 0.5618 | 0.149 | 1.890 | +15.7 |
| Zinc | 0.2665 | 0.4947 | 0.133 | 1.856 | +13.6 |
| Ideal HCP | 1.633 | 0 | |||
| Magnesium | 0.3209 | 0.5209 | 0.160 | 1.623 | -0.66 |
| Cobalt | 0.2507 | 0.4069 | 0.125 | 1.623 | -0.66 |
| Zirconium | 0.3231 | 0.5148 | 0.160 | 1.593 | -2.45 |
| Titanium | 0.2950 | 0.4683 | 0.147 | 1.587 | -2.81 |
| Beryllium | 0.2286 | 0.3584 | 0.113 | 1.568 | -3.98 |
So materials that are called “close-packed” are really named because they are a collection of close-packed layers, rather than having a specific packing factor.
How do you write close-packed structures?
If you’re doing a PhD in crystallography and you need to learn different types of notation, Krishna and Pandey have an excellent article here, which I will try to summarize for those who aren’t doing their PhD in crystallography.
Final Thoughts
Generally speaking, close-packed crystals are made by stacking 2-dimensional, hexagonal close-packed layers on top of each other. To pack the planes at tightly as possible in the 3rd dimension, these planes can be in one of three orientations, which we call A, B, and C.
Due to quantum mechanics and atomic interactions, crystals do not form with a random arrangement of these 3 layers. Instead, the order of these layers is fixed, and defines the crystal structure. For example, the stacking order ABC-ABC results in an FCC crystal, while the stacking order AB-AB results in an HCP crystal.
There can be defects in the stacking order, such as a stacking fault. Stacking faults are when there is an extra plane, or a missing plane.
The four types of close-packed crystals that occur at STP in pure elements are: FCC, HCP, DHCP, and Sm-type (close packed rhombohedral).
References and Further Reading
[1] Meric de Bellefon, Gabriel, Influence of Deformation Twins on the Radiation Response of 316 Austenitic Stainless Steel, 2018, 10.13140/RG.2.2.23810.79042.
[2] P. Krishna and D. Pandey, Close-Packed Structures, International Union of Crystallography, 1981
For a detailed, technical look at the crystallography behind close-packed structure’s check out Krishna and Pandey’s chapter.
As always a great resource from Professor Föll. This page links to a section about stacking faults, but you can find a wealth of materials science knowledge in his website.
If you’re interested in this very technical article about close-packed crystals, you may be interested in our other crystallography articles (in recommended reading order).
Introduction to Bravais Lattices
What is the Difference Between “Crystal Structure” and “Bravais Lattice”
Atomic Packing Factor
How to Read Miller Indices
How to Read Hexagonal Miller-Bravais Indices
Close-Packed Crystals and Stacking Order
Interstitial Sites
Primitive Cells
How to Read Crystallography Notation
What are Point Groups
List of Point Groups
What are Space Groups
List of Space Groups
The 7 Crystal Systems
If you are interested in more details about any specific crystal structure, I have written individual articles about simple crystal structures which correspond to each of the 14 Bravais lattices:
1. Simple Cubic
2. Face-Centered Cubic
2a. Diamond Cubic
3. Body-Centered Cubic
4. Simple Hexagonal
4a. Hexagonal Close-Packed
4b. Double Hexagonal Close-Packed (La-type)
5. Rhombohedral
5a. Rhombohedral Close-Packed (Sm-type)
6. Simple Tetragonal
7. Body-Centered Tetragonal
7a. Diamond Tetragonal (White Tin)
8. Simple Orthorhombic
9. Base-Centered Orthorhombic
10. Face-Centered Orthorhombic
11. Body-Centered Orthorhombic
12. Simple Monoclinic
13. Base-Centered Monoclinic
14. Triclinic